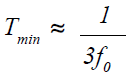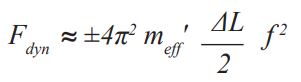Resonant Frequency
The resonant frequencies specified for longitudinal stack actuators apply to operation when not clamped on both sides. In an arrangement with unilateral clamping, the value has to be divided in half.
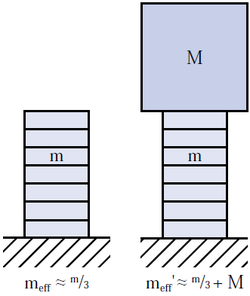
The reducing influence of an additional load on the resonant frequency can be estimated with the following equation (for the calculation of meff see fig. 1):
![Resonant frequency with load [Hz] Resonant frequency with load [Hz]](/fileadmin/user_upload/pi_ceramic/IMG/PIC_Gleichung_17.png)
f0' |
|
| f0 | Resonant frequency without load [Hz] |
| meff | Effective mass of the piezo stack actuator [kg] |
| meff' | Effective mass of the piezo stack actuator with load [kg] |
| φ | Phase angle [degrees] |
| f | Control frequency [Hz] |
| m | Mass of the piezo actuator |
| M | Additional load |
| Tmin | Time in which the piezo actuator can reach its nominal displacement. |
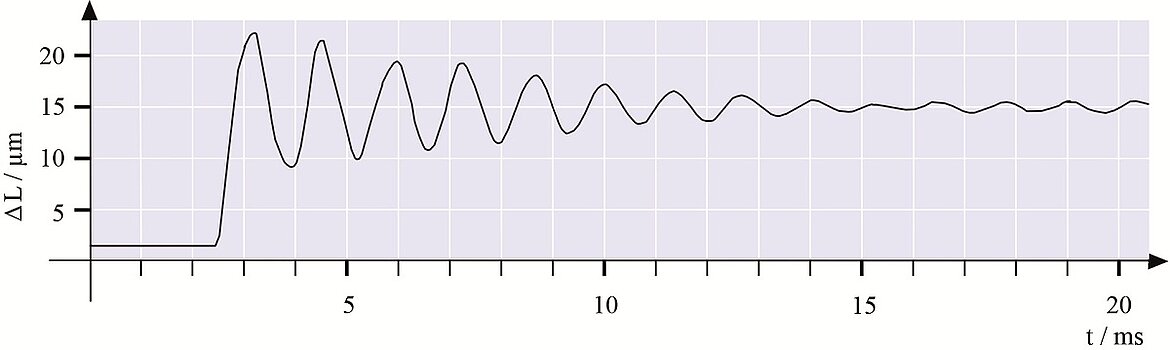
Fast response behavior is a characteristic feature of piezo actuators. A fast change in the operating voltage causes a fast position change. This behavior is desired especially in dynamic applications, such as scanning microscopy, image stabilization, valve controls, generating shock waves, or active vibration damping. When the control voltage suddenly increases, a piezo actuator can reach its nominal displacement in approximately one third of the period of its resonant frequency f0 (fig. 2):
With suitable drive electronics, piezo actuators can generate high accelerations of several ten thousand m/s². As a result of the inertia of possible coupled masses and of the actuators themselves, dynamic pull forces occur, which have to be compensated for with >> Mechanical Preloads. In sinusoidal operation, the maximum forces can be estimated as follows:
![Phase angle [degrees] Phase angle [degrees]](/fileadmin/user_upload/pi_ceramic/IMG/PIC_Gleichung_18.png)